Draw A Quadrilateral That Has At Least 1 Right Angle
Draw A Quadrilateral That Has At Least 1 Right Angle - They rotate, too!so you can become familiar with them from all angles Parallel sides and right angles in a quadrilateral. And it's an interesting proof. Web every quadrilateral can be decomposed (cut apart) into two triangles, each of whose angles' measures sum to 180º. This has 4 sides and 4 angles but the angles are not all right angles. You would find that for every quadrilateral, the sum of the interior angles will always be. Web every triangle you can draw on the surface of the earth has an angle sum strictly greater than 180°. Students who know the analogous result for triangles can convince themselves of this by. A convex quadrilateral is a polygon with all interior angles less than 180^ {\circ}. It just needs 4 angles. Web every triangle you can draw on the surface of the earth has an angle sum strictly greater than 180°. Web you could draw many quadrilaterals such as these and carefully measure the four angles. Maybe i'll do a video. Web quadrilaterals can be convex or concave. Identify properties, including angle measurements, of quadrilaterals. Web you could draw many quadrilaterals such as these and carefully measure the four angles. Maybe i'll do a video. Read about quadrilaterals, and then play with them here. In fact, you can draw a triangle on the earth that has three. Parallel sides and right angles in a quadrilateral. Quadrilaterals are a special type of polygon. Parallel sides and right angles in a quadrilateral. Web the main shapes are square, rectangle, rhombus, kite, parallelogram, and trapezoid. They rotate, too!so you can become familiar with them from all angles Web so for example, this interior angle right over here is larger than 180 degrees. A quadrilateral with only 2 right angles. It just needs 4 angles. Web so for example, this interior angle right over here is larger than 180 degrees. You would find that for every quadrilateral, the sum of the interior angles will always be. It's actually a pretty simple proof to show that, if you have a concave quadrilateral, if at. You would find that for every quadrilateral, the sum of the interior angles will always be. A convex quadrilateral is a polygon with all interior angles less than 180^ {\circ}. The sum of the interior angles in a quadrilateral is 360°. Web the main shapes are square, rectangle, rhombus, kite, parallelogram, and trapezoid. Maybe i'll do a video. Read about quadrilaterals, and then play with them here. They rotate, too!so you can become familiar with them from all angles You would find that for every quadrilateral, the sum of the interior angles will always be. A convex quadrilateral is a polygon with all interior angles less than 180^ {\circ}. Web the main shapes are square, rectangle, rhombus, kite,. As with triangles and other. So with some logic you can see that if one line a is. It just needs 4 angles. Web the diefinition of right angle is a measure of 90 deg, which means the two lines are perpendicular to each other. Web no, a quadrilateral does not need to have 4 right angles. Web angles in a quadrilateral. Students who know the analogous result for triangles can convince themselves of this by. A convex quadrilateral is a polygon with all interior angles less than 180^ {\circ}. Web you could draw many quadrilaterals such as these and carefully measure the four angles. Web quadrilaterals can be convex or concave. You would find that for every quadrilateral, the sum of the interior angles will always be. Parallel sides and right angles in a quadrilateral. Web you could draw many quadrilaterals such as these and carefully measure the four angles. Web the main shapes are square, rectangle, rhombus, kite, parallelogram, and trapezoid. It's actually a pretty simple proof to show that,. Quadrilaterals are a special type of polygon. So with some logic you can see that if one line a is. Web no, a quadrilateral does not need to have 4 right angles. Identify properties, including angle measurements, of quadrilaterals. It's actually a pretty simple proof to show that, if you have a concave quadrilateral, if at least one of the. And it's an interesting proof. Students who know the analogous result for triangles can convince themselves of this by. They rotate, too!so you can become familiar with them from all angles Web quadrilaterals can be convex or concave. Web the main shapes are square, rectangle, rhombus, kite, parallelogram, and trapezoid. Web angles in a quadrilateral. A convex quadrilateral is a polygon with all interior angles less than 180^ {\circ}. Maybe i'll do a video. Web you could draw many quadrilaterals such as these and carefully measure the four angles. It's actually a pretty simple proof to show that, if you have a concave quadrilateral, if at least one of the interior angles has a measure larger. The sum of the interior angles in a quadrilateral is 360°. Web every triangle you can draw on the surface of the earth has an angle sum strictly greater than 180°. Read about quadrilaterals, and then play with them here. Web so for example, this interior angle right over here is larger than 180 degrees. A parallelogram is a quadrilateral with 2 pair of opposite sides. Quadrilaterals are a special type of polygon.
Show Me Pictures Of Quadrilateral Shapes picshenanigan

How To Draw A Quadrilateral
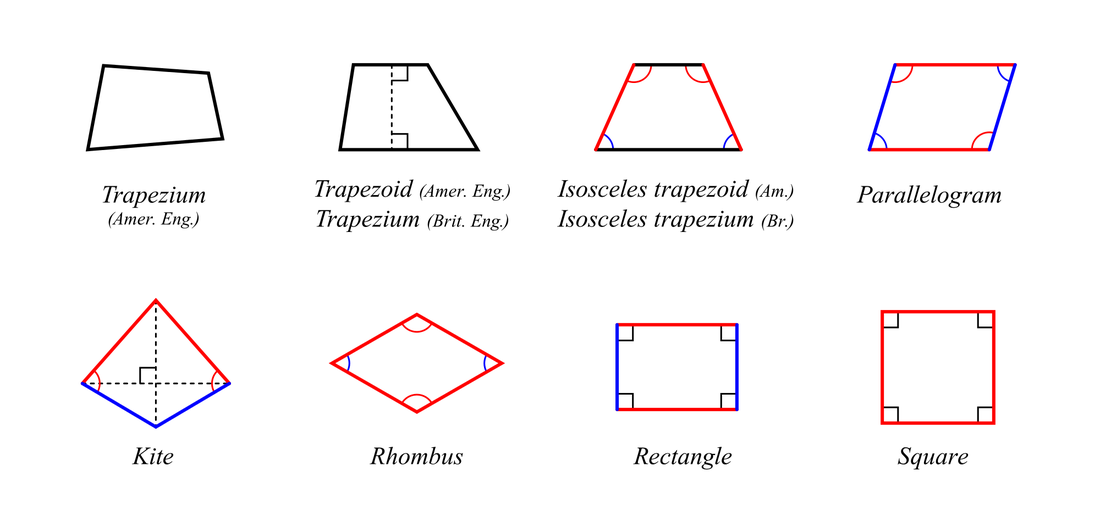
Properties of a Quadrilateral Grade 10 principles of mathematics

Quadrilaterals, polygons and transformations (PreAlgebra, Introducing
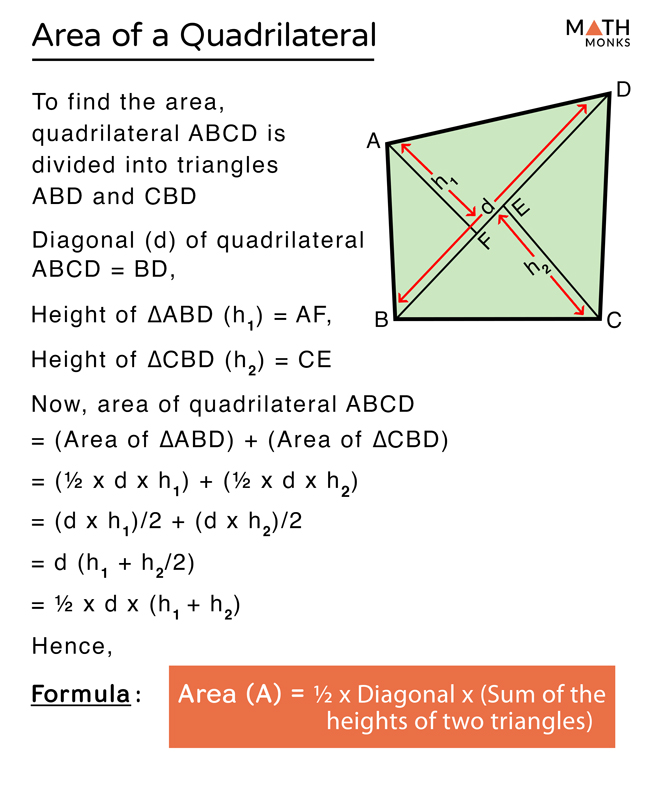
Understanding Quadrilaterals Class 8 Formulas
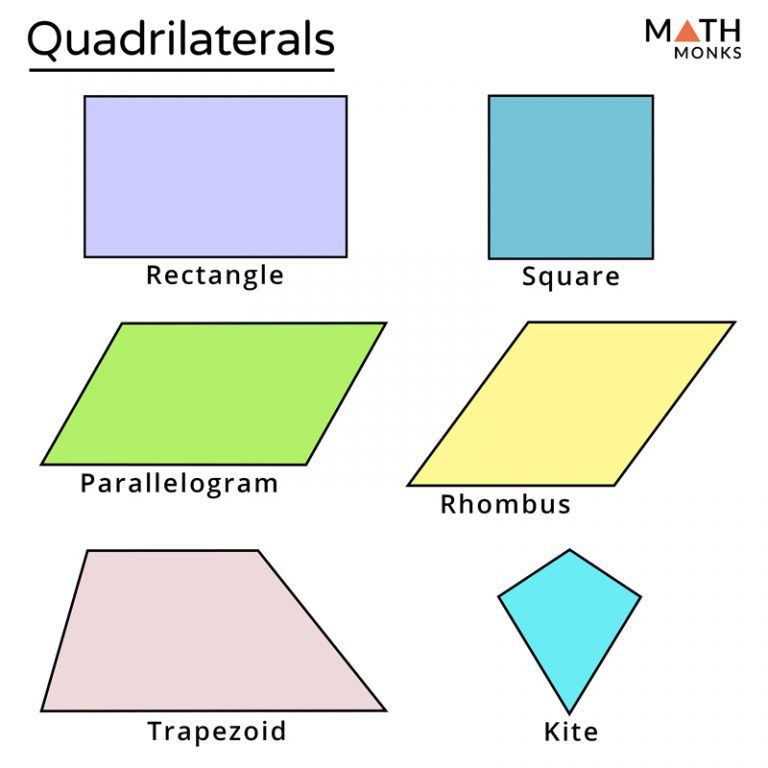
Quadrilateral Definition, Properties, Types, Formulas, Examples
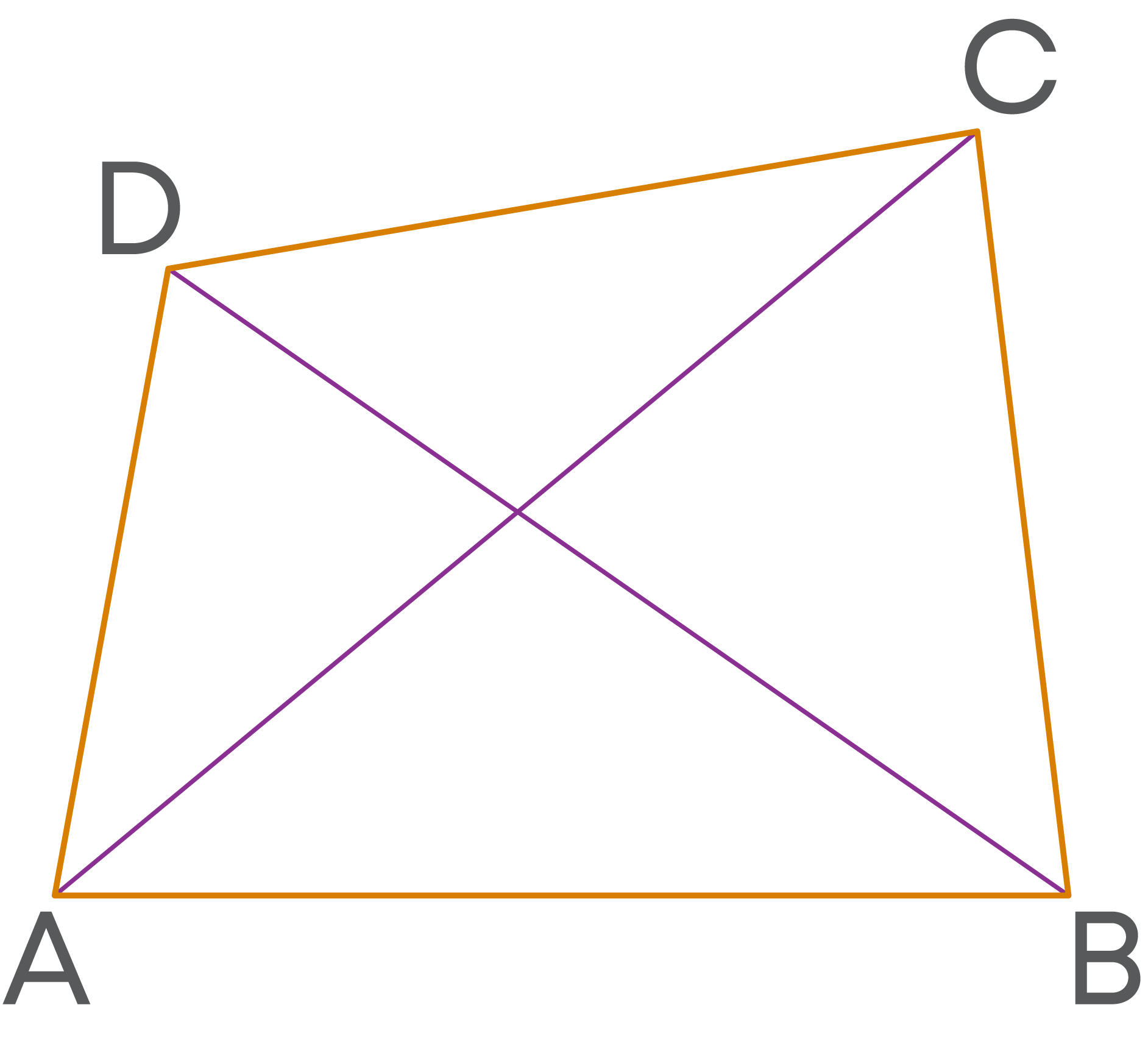
Understanding Quadrilaterals 8 Blog

Quadrilaterals Teaching math strategies, Learning mathematics, Math

Draw a quadrilateral that has exactly one right angle. Quizlet
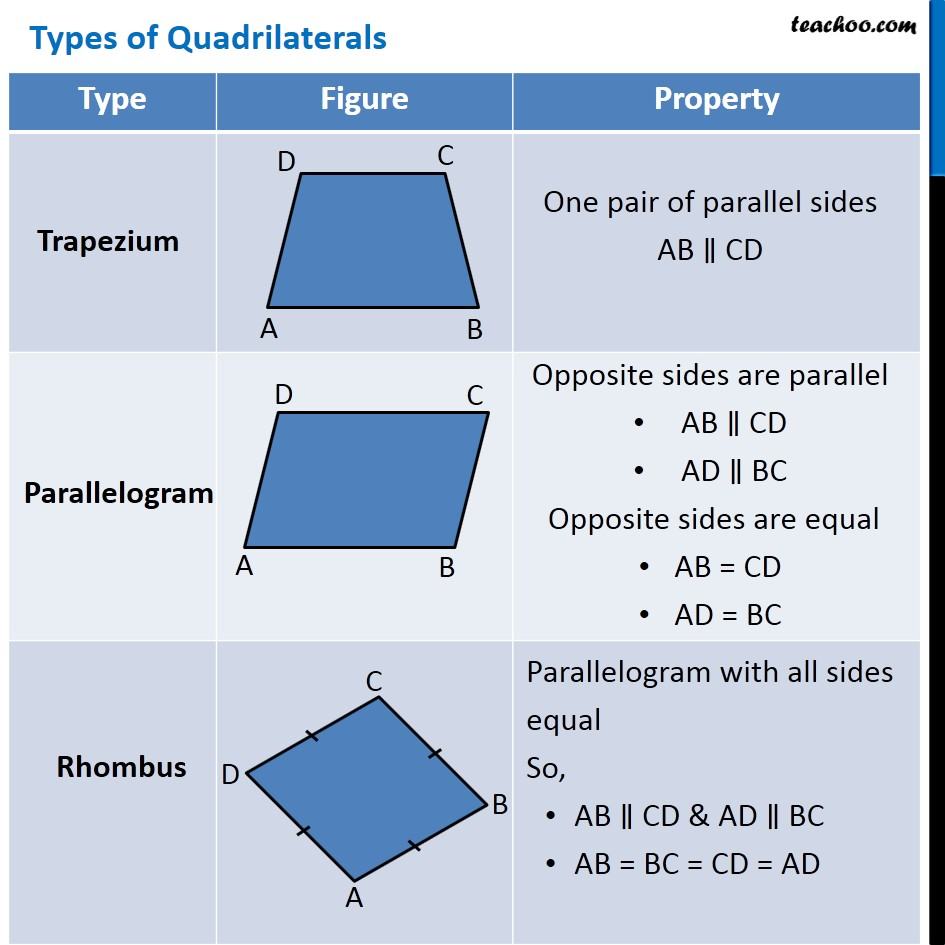
Types of Quadrilaterals and their properties Teachoo Types of Quad
In Fact, You Can Draw A Triangle On The Earth That Has Three.
Web No, A Quadrilateral Does Not Need To Have 4 Right Angles.
Parallel Sides And Right Angles In A Quadrilateral.
So With Some Logic You Can See That If One Line A Is.
Related Post: